| Title | Optimal quantizers for Radon random vectors in a Banach space |
| Publication Type | Journal Article |
| Year of Publication | 2007 |
| Authors | Siegfried Graf, Harald Luschgy, and Gilles Pagès |
| Journal | J. Approx. Theory |
| Volume | 144 |
| Pagination | 27–53 |
| ISSN | 0021-9045 |
| Abstract | For every integer |
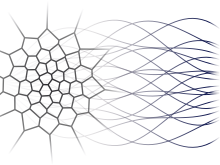
 and evrery positive real number
and evrery positive real number 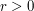 and a Radon random vector
and a Radon random vector 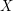 with values in a Banach space
with values in a Banach space 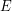 , let
, let ![$ e_{n,r}(X,E) = \inf{\left(E\left[\min\limits_{a \in \alpha} \| X-a \|^r \right]^{1/r}\right)} $](/sites/default/files/tex/83956ac247021abb2dffed2a58a1f885ce170b04.png) , where the infimum is taken over all subsets
, where the infimum is taken over all subsets  of
of 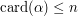 (
(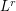 -quantization problem, derive their stationarity properties and establish for
-quantization problem, derive their stationarity properties and establish for 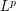 -spaces
-spaces 