| Title | Functional quantization of a class of Brownian diffusions: a constructive approach |
| Publication Type | Journal Article |
| Year of Publication | 2006 |
| Authors | Harald Luschgy, and Gilles Pagès |
| Journal | Stochastic Processes and their Applications |
| Volume | 116 |
| Pagination | 310 - 336 |
| ISSN | 0304-4149 |
| Keywords | Brownian diffusions, functional quantization, Lamperti transform; Girsanov theorem, optimal quantizers |
| Abstract | The functional quantization problem for one-dimensional Brownian diffusions on |
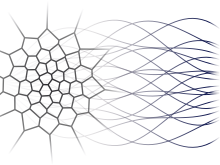
![$ [0,T] $](/sites/default/files/tex/b9baa7710b108fe1c29bd70224f83e1ccc813636.png) is investigated. One shows under rather general assumptions that the rate of convergence of the
is investigated. One shows under rather general assumptions that the rate of convergence of the 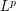 -quantization error is
-quantization error is 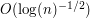 like for the Brownian motion. Several methods to construct some rate-optimal quantizers are proposed. These results are extended to
like for the Brownian motion. Several methods to construct some rate-optimal quantizers are proposed. These results are extended to 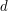 -dimensional diffusions when the diffusion coefficient is the inverse of a gradient function. Finally, a special attention is given to diffusions with a Gaussian martingale term.
-dimensional diffusions when the diffusion coefficient is the inverse of a gradient function. Finally, a special attention is given to diffusions with a Gaussian martingale term.