We investigate the connections between the mean pathwise regularity of stochastic processes and their 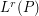 -functional quantization rates as random variables taking values in some -functional quantization rates as random variables taking values in some ![$ L^p([0,T],dt) $](/sites/default/files/tex/64d8adafc7e2051e336141b47740a50615d549d4.png) -spaces ( -spaces (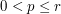 ). Our main tool is the Haar basis. We then emphasize that the derived functional quantization rate may be optimal (e.g., for Brownian motion or symmetric stable processes) so that the rate is optimal as a universal upper bound. As a first application, we establish the ). Our main tool is the Haar basis. We then emphasize that the derived functional quantization rate may be optimal (e.g., for Brownian motion or symmetric stable processes) so that the rate is optimal as a universal upper bound. As a first application, we establish the 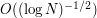 upper bound for general Itô processes which include multidimensional diffusions. Then, we focus on the specific family of Lévy processes for which we derive a general quantization rate based on the regular variation properties of its Lévy measure at upper bound for general Itô processes which include multidimensional diffusions. Then, we focus on the specific family of Lévy processes for which we derive a general quantization rate based on the regular variation properties of its Lévy measure at  . The case of compound Poisson processes, which appear as degenerate in the former approach, is studied specifically: we observe some rates which are between the finite-dimensional and infinite-dimensional "usual'' rates . The case of compound Poisson processes, which appear as degenerate in the former approach, is studied specifically: we observe some rates which are between the finite-dimensional and infinite-dimensional "usual'' rates | 
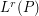 -functional quantization rates as random variables taking values in some
-functional quantization rates as random variables taking values in some ![$ L^p([0,T],dt) $](/sites/default/files/tex/64d8adafc7e2051e336141b47740a50615d549d4.png) -spaces (
-spaces (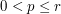 ). Our main tool is the Haar basis. We then emphasize that the derived functional quantization rate may be optimal (e.g., for Brownian motion or symmetric stable processes) so that the rate is optimal as a universal upper bound. As a first application, we establish the
). Our main tool is the Haar basis. We then emphasize that the derived functional quantization rate may be optimal (e.g., for Brownian motion or symmetric stable processes) so that the rate is optimal as a universal upper bound. As a first application, we establish the 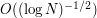 upper bound for general Itô processes which include multidimensional diffusions. Then, we focus on the specific family of Lévy processes for which we derive a general quantization rate based on the regular variation properties of its Lévy measure at
upper bound for general Itô processes which include multidimensional diffusions. Then, we focus on the specific family of Lévy processes for which we derive a general quantization rate based on the regular variation properties of its Lévy measure at  . The case of compound Poisson processes, which appear as degenerate in the former approach, is studied specifically: we observe some rates which are between the finite-dimensional and infinite-dimensional "usual'' rates
. The case of compound Poisson processes, which appear as degenerate in the former approach, is studied specifically: we observe some rates which are between the finite-dimensional and infinite-dimensional "usual'' rates