| Title | The high resolution vector quantization problem with Orlicz norm distortion |
| Publication Type | Journal Article |
| Year of Publication | 2010 |
| Authors | Steffen Dereich, and Christian Vormoor |
| Keywords | complexity, discrete approximation, High-resolution quantization, self similarity |
| Abstract | Summary. We derive a high-resolution formula for the quantization problem under Orlicz norm distortion. In this setting, the optimal point density solves a variational problem which comprises a function |
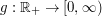 characterizing the quantization complexity of the underlying Orlicz space. Moreover, asymptotically optimal codebooks induce a tight sequence of empirical measures. The set of possible accumulation points is characterized and in most cases it consists of a single element. In that case, we find convergence as in the classical setting.
characterizing the quantization complexity of the underlying Orlicz space. Moreover, asymptotically optimal codebooks induce a tight sequence of empirical measures. The set of possible accumulation points is characterized and in most cases it consists of a single element. In that case, we find convergence as in the classical setting.