Home » Publications

Méthodes de quantification optimale avec applications à la finance

TitleMéthodes de quantification optimale avec applications à la finance
Publication TypeThesis
Year of Publication2008
AuthorsAbass Sagna
AdvisorGilles Pagès
Keywordsbarrier options, Lloyd algorithm, lookback options, maximal radius, quantization, rate-optimal
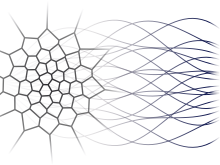
Abstract

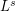This thesis is dovoted to optimal quantization with some applications to mathematical finance. Chap.1 reminds the bases of optimal quantization and numerical search of optimal quantizers. In chap.2 we study the asymptotics, in $ L^s $, of the quantization error associated to a linear transform of an 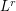$ L^r $ optimal sequence of quantizers. We show that such a transformation allows to make the transformed sequence 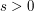$ L^s $ rate optimal for every $ s>0 $, for a large family of probabilities. Chap.3 deals with the asymptotics of the maximal radius sequence associated to an 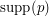$ L^r $ optimal sequence of quantizers. We show that as soon as $ \textrm{supp}(p) $ is unbounded, the maximal radius converge to infinity. We then give the rate of convergence for a large family of probabilities. Chap.4 is devoted to the pricing of lookback and barrier like options. We write these prices in a form which allows us to estimate them by Monte-Carlo, by an hybrid Monte-Carlo-quantization and by a pure quantization method.

Native Abstract

Cette thèse est consacrée à la quantification avec des applications à la finance. Le chap.1 rappelle les bases de la quantification et les méthodes de recherche de quantifieurs optimaux. Au chap.2 on étudie le comportement asymptotique, dans $ L^s $, de l'erreur de quantification associée à une transformation linéaire d'une suite de quantifieurs optimale dans $ L^r $. On montre qu'une telle transformation permet de rendre la suite transformée $ L^s $ taux optimale pour tout $ s $, pour une large famille de probabilités. Le chap.3 étudie le comportement asymptotique de la suite du rayon maximal associée à une suite de quantifieurs $ L^r $ optimale. On montre que dès que $ \textrm{supp}(p) $ est non borné cette suite tend vers l'infini. On donne, pour une grande famille de probabilités, la vitesse de convergence vers l'infini. Le chap.4 est consacré au pricing d'options de type lookback et à barrièrre. On écrit ces prix sous une forme qui nous permet de les estimer par Monte-Carlo, par une méthode hybride Monte-Carlo-quantification et par pure quantification.